
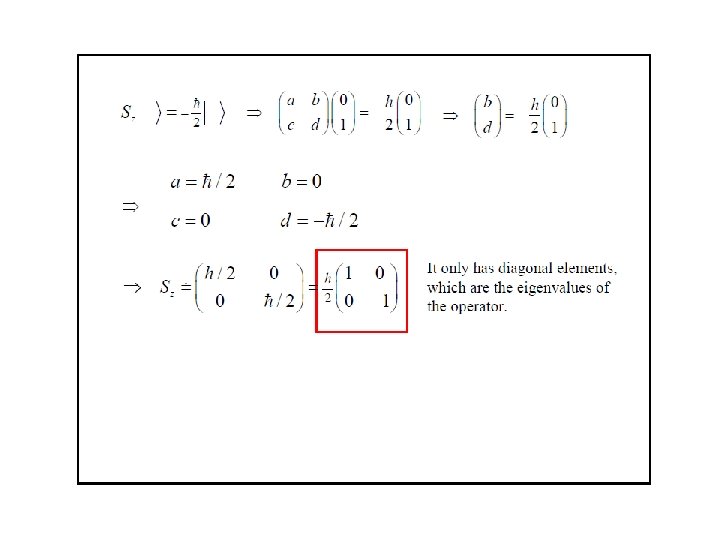
Your Hamiltonian doesn’t give you a clean unitary operator that can perfectly describe the mechanics of the system under observation. This implies your formal ideas about unitary evolution (postulate 2) isn’t very correct. The postulates are applicable on closed, isolated systems.Īnd simply put, this is hardly a case ever in the real world. I would rather discuss a bigger picture and hope it will make the distinction between general and projective measurements much clearer. I’ll refrain from straightaway launching the formal discussion on measurements here. Without going into deeper math, anything of the form exp(-i.K) where K is a Hermitian operator yields a Unitary operator. Multiplication of a Hermitian operator by a scalar (which is essentially the ratio of difference of time and Dirac-h) preserves the Hermitian property. The term inside the exp part requires more explanation. Given a closed quantum system, the evolution of the system is described with a unitary transformation. Read more about Hilbert spaces here Postulate 2 This allows several operations like measurement of length and angles. Intuitively, such a space is described to combine Euclidean (or mainly 2-dimensional spaces) calculus and notions of linear algebra to multi-dimensional spaces. In simple terms, if you have an isolated quantum system, there is a complex vector space with an inner product defined attached to that system, called the state space. I’ll begin with the pre-requisite postulates of quantum mechanics: Postulate 1 You’ll ask questions and understand better that way (and that is specifically true for the entire article). Interpret my statements as a sort of quantum statements (a word I coined just now )with a certain degree of uncertainty attached to them. Wait! Didn’t I say two kinds of measurements and named three. When I finally fit the different pieces together, I thought it would be helpful to carve out a different approach to understanding quantum measurements (a kind of top-bottom approach - and that means reserving the math to the last while understanding the broader implications first) and first understanding why do we need a distinction between the two kinds of measurements I shall discuss here- general and projection and POVM. The math wasn’t that tough, but the basic intuition of why doing the things the way they are done was missing, at least in my source. When I set out to learn about quantum measurements, things were not very smooth. Let’s get to the point! Why this article?
Also, consult other sources about (complex) vector spaces, inner products, linear combinations, and related concepts.įine then, pre-requisites done. If you are facing difficulty in understanding terms like qubit, superposition, basis vectors, I’ll suggest reading this article as an introduction, the bra and ket notation, and this linear algebra review (and do not leave out Eigenvectors and Eigenspaces, orthogonality). On a top level, measurement is essentially what the figure above depicts: some operation on a qubit (some sort of superposition state of basis vectors|0> and |1>) to get a classical bit (the process of which is completely random).īefore you begin. The cat is shown in that probability space as a vector with equal components a and d.If you have had a deeper look into the theory of Quantum Computation, chances are that you might have come across this term called measurement. The basis is the two vectors alive and dead. It says the state of the cat is in a superposition of the two states "alive" and "dead". There is an equal chance of it being alive or dead (until we open the box). So yes it is an orthonormal basis! Schrödinger's CatĪ famous example is "Schrödinger's Cat": a thought experiment where a cat is in a box with a quantum-triggered container of gas. Our simple example from above works nicely: Normalized: each basis vector has length 1.We can test it by making sure any pairing of basis vectors has a dot product a Orthogonal: each basis vector is at right angles to all others.In most cases we want an orthonormal basis which is: Matrix Rank has more details about linear dependence, span and more. In this case they are simple unit vectors, butĪny set of vectors can be used when they are independent of each other (being at right angles achieves this) and can together span every part of the space. The vectors "1, 0, 0", "0, 1, 0" and "0, 0, 1" form the basis: the vectors that we


 0 kommentar(er)
0 kommentar(er)
